By : Varsha Deiveegan
This is Part 1 of “Interest”. Click here for Part II.
Simple and Compound Interest form an important part of maths that is required to be learnt for CLAT. Simple Interest is actually very simple! But compound interest can get you a little apprehensive because of the huge numbers and the ‘power’ factor. But it is easy provided you use your calculation skills and since there is time constraint, speed becomes very very important. We’ll use certain shortcuts to ace this and make compound interest simple!
Let’s start with defining some terms that are common to both compound and simple interest.
Interest– Interest is the price paid by the borrower for the use of a lender’s money. If you borrow a certain some of money for a particular period of time, you would pay more money than the money you actually borrowed. This ‘extra’ sum of money is nothing but the interest. For example, in the month of January you borrow Rs.2,500. In the next January, you pay 5000. So, the interest here is Rs.3,500- Rs.2,500 i.e. Rs.1,000.
Principal– Principal is the initial value of lending. Say, you invest; in this case, the value of initial investment is also called the principal. Suppose, you borrow or lend Rs.2,500, this is your principal.
Rate of Interest– The rate at which interest is charged for a defined length of time for use of principal is called the rate of interest. It is usually expressed as a percentage. For instance, if you invest Rs.20,000 in your bank account for one year, the rate of interest being 5% per annum. It simply means, you will earn Rs.5 as interest for every Rs.100 of principal amount in a year.
Accumulated Amount– It is the sum total of principal and interest. For example, you deposit Rs.50,000 in a bank for one year with 5% rate of interest per annum; you would earn an interest of Rs.2,500 after a year. So, the amount at the end of one year is Rs.50,000 + Rs.2,500 = Rs.52,500.
SIMPLE INTEREST
In simple interest, the principal remains the same for calculating interest for the entire period of borrowing. So, it is not calculated on interest previously earned. This trickles down to the thumb rule of simple interest- No interest is paid on interest earned during the term of loan and the base principal remains constant.
It is calculated as follows-
If ‘P’ is the principal, ‘R’, the ROI and ‘T’ is the time period, then
I = PRT/ 100 ………. (1)
Also, Amount ‘A’ = P + I = P + PTR/ 100 = P ( 1 + TR/100) ………. (2)
So, out of the four variables I, P, R, and T, if three are given, the other one can be found out. But don’t expect such questions in CLAT.
Illustration 1: In what time will Rs.1250 amount to Rs.2150 at a 9% p.a SI?
- Here as we can infer Rs. 2150 is the amount and Rs.1250 is the principal. ROI = 9%.
- 2150 = 1250 [ 1 + (9T)/100] ……. Using (2)
- 2150/1250 = 1 + 9T/100
- (0.72 * 100)/9 = T
- T = 8 years
Illustration 2: A sum of money at simple interest amounts to Rs.815 in 3 years and to Rs.854 in 4 years. Find the sum.
- We know that A = P + I
- P + S.I. for 4 years = Rs.854 …… (i)
- P + S.I. for 3 years = Rs.815 …… (ii)
- Subtracting (ii) from (i),
- S.I. for 1 year = Rs.39
- S.I. for 3 years = Rs.39 * 3 = Rs.117 …… (iii)
- Subtracting (iii) from (ii), ……. Using- S.I.- A = P
- P/ sum = Rs.815 – Rs.117 = Rs.698
Shortcuts-
1. A sum of money will double itself in 100/ R years.
Illustration 1: A sum of money amounts to Rs. 3567 in 4.67 years at 10.5% SI.
When will it double itself at the same rate?
- Going by the above shortcut, it is 100/ 10.5 = 9.52 years
2. If a sum of money becomes n1 times in T1 years and n2 times in T2 years, then the ratio of the times will be given by
T1/ T2 = (n1-1)/ (n2-1)
This can be used to find out when a sum will treble.
Illustration 2: A sum of money doubles itself in 8 years. In how many years will it treble itself?
- n1 = 2, n2 = 3 and T1 = 8, T2 = ?( say x)
- 8/ x = ½
- x = 16
Illustration 3: A sum of money trebles itself in 8 years. In how many years will it Become five times? SI being reckoned.
- n1 = 3, n2 = 5 and T1= 8, T2= x
- 8/ x = 2/ 4
- x = 16
3. The rate at which a sum becomes double in a given time period, ‘n’, is given by the formula, 100/n.
PRACTICE!! : There are 10 Questions below. Select an option and click “Next”. At the end, answers and explanations will be displayed.
This is Part 1 of “Interest”. Click here for Part II.







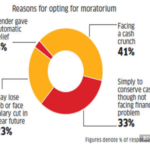


thax for supplement…..
it was not that easy and i got 8 right but took 10 min.
Rishabh,
The SI qns can be easily done within 10 mins..it just takes practice..try n work out math qns in as less time as possible..
can u provide me with explanation for Q 5 and Q 6…
Full marks ! Unbelievable !!!!! :O :O :O
can you provide me for q7 q 9 plzzzzzzzzzzzz
i cldnt understand question no 6 n 7..plz if u cld help..?
i think it is not treble and it is triple…!!!
can i get the method ie the answers method for the above exercise..?
may i please have the explanation for question 6?
These were pretty easy and fun 🙂
WOW, THANKS! the tricks are like real great, but i was hoping for explanations to each of these questions :/
can i get the solutions for the above exercise
hoping a reply soon .